Orthogonalité et distances dans l’espace

Pour tout ce qui suit, on munit l'espace d'un repère orthonormé $(\mathrm{O} ; \vec{i} ; \vec{j} ; \vec{k})$.

Expression analytique du produit scalaire dans un repère orthonormé

Pour $\vec{u}(x ; y ; z)$ et $\vec{v}(x' ; y' ; z')$, deux vecteurs de l'espace :

$$\vec{u} \cdot \vec{v} = xx' + yy' + zz'$$

qui est un nombre réel.

Exemple

Pour $\vec{u}(2~;~-1~;~3)$, $\vec{v}(1~;~0~;~-2)$, deux vecteurs :

Calcul

$$\vec{u} \cdot \vec{v} = 2 \times 1 + (-1) \times 0 + 3 \times (-2) = -4$$

Propriétés du produit scalaire

Pour $\vec{u}$, $\vec{v}$ et $\vec{w}$ trois vecteurs de l'espace et un nombre réel $k$ :

• Commutativité : $\vec{u} \cdot \vec{v} = \vec{v} \cdot \vec{u}$
• Associativité avec un scalaire : $(k\vec{u}) \cdot \vec{v} = \vec{u} \cdot (k \vec{v}) = k(\vec{u} \cdot \vec{v})$

EN RÉSUMÉ

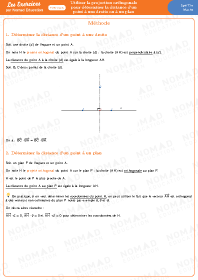
Norme d'un vecteur

Pour $\vec{u}(x~;~y~;~z)$ un vecteur de l'espace, la norme se calcule selon la formule suivante :

$$\| \overrightarrow u \| = \sqrt{x^2 + y^2 + z^2}$$

Distance entre deux points

Pour $\mathrm{A }({x}_{\mathrm{A}} ; {y}_{\mathrm{A}} ; {z}_{\mathrm{A}})$ et $\mathrm{B}({x}_{\mathrm{B}} ; {y}_{\mathrm{B}} ; {z}_{\mathrm{B}})$, deux points de l'espace, la distance entre ces points est donnée par :

$$\mathrm{AB} = \sqrt{\overrightarrow{\mathrm{AB}} \cdot \overrightarrow{\mathrm{AB}}} = \sqrt{{({x}_{\mathrm{B}} - {x}_{\mathrm{A}})}^2 + {({y}_{\mathrm{B}} - {y}_{\mathrm{A}})}^2 + {({z}_{\mathrm{B}} - {z}_{\mathrm{A}})}^2}$$

Exemple

Pour les points $\mathrm{A}(-1~;~ -2 ~; ~2)$ et $\mathrm{B}(3~; ~0~ ;~ 1)$, nous avons $\overrightarrow{\mathrm{AB}}(4~;~2~;~-1)$ et :

$$\mathrm{AB} = \sqrt{4^2+2^2+{(-1)}^2} = \sqrt{16+4+1} = \sqrt{21}$$

Vecteurs orthogonaux

Deux vecteurs de l'espace sont orthogonaux si et seulement si leur produit scalaire est nul.

Exemple

Les vecteurs $\vec{u}(2~;~-1~; ~3)$ et $\vec{v}(2~;~4~;~0)$ sont orthogonaux car :

$$\overrightarrow{u} \cdot \overrightarrow{v} = 2 \times 2 + (-1) \times 4 + 3 \times 0 = 4 - 4 = 0$$

EN RÉSUMÉ

Vecteur normal à un plan

Définition du vecteur normal

Un vecteur $\vec{n}$ non nul de l'espace est un vecteur normal à un plan $\rm P$ s'il est orthogonal à tous les vecteurs du plan $\rm P$, donc à au moins deux vecteurs non colinéaires du plan $\rm P$.

Caractérisation d'un plan par son vecteur normal

Le plan $\rm P$ passant par le point $\rm A$ et de vecteur normal non nul $\vec{n}$ est donc l'ensemble des points $\rm M$ tels que :

$\overrightarrow{\mathrm{AM}}$ et $\vec{n}$ sont orthogonaux, soit $\overrightarrow{\mathrm{AM}} \cdot \vec{n} = 0$.

EN RÉSUMÉ

En partenariat avec